Sin 2x, Cos 2x, Tan 2x is the trigonometric formulas which are called as double angle formulas because they have double angles in their trigonometric functions Let's understand it by practicing it through solved example Introduction to Tan double angle formula2319Proving the trigonometric identity $(\tan{^2x}1)(\cos{^2(x)}1)=\tan{^2x}$ has been quite the challenge I have so far attempted using simply the basic trigonometric identities based on the Pythagorean Theorem I am unsure if these basic identities are unsuitable for the situation or if I am not looking at the right angle to tackle this problemVerify the Identity tan(2x)^2sin(2x)^2cos(2x)^2=sec(2x)^2 Start on the left side Apply Pythagorean identity Tap for more steps Apply pythagorean identity Apply pythagorean identity Because the two sides have been shown to be equivalent, the equation is an identity is an identity

1 Tan 2x 1 Tan 2 X 1 2cos 2 X Youtube
Tan 2x identity proof
Tan 2x identity proof-Identities related to sin 2x, cos2x, tan 2x, sin3x, cos3x, and tan3x Sin 2x = Sin 2x = sin(2x)=2sin(x) cos(x) Sin(2x) = 2 * sin(x)cos(x) Proof To express Sine, the formula of "Angle Addition" can be usedTanx = t Sec^2 x dx= dt So now it is, 1/ (1t)^2 dt This integral is given by 1/1t and t= tanx So, it is cosx/cosx sinx tanx = t Sec^2 x dx= dt So now it is, 1/ (1t)^2 dt This integral is given by 1/1t and t= tanx So, it is cosx/cosx sinx Integral of the function \frac {\cos ^2 x} {1\tan x}
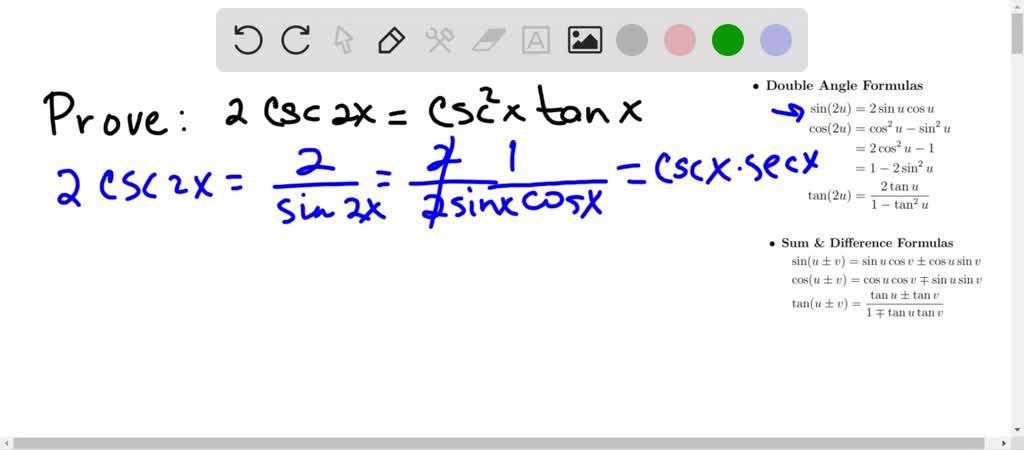


Solved In Exercises 15 22 Prove The Identity 2
Sum and Difference Identities For the exercises 14, find the exact value 1) \(\tan \left (\dfrac{7\pi }{12} \right )\) Answer \(2\sqrt{3}\)In this video, we are going to derive the identity for the tangent of 2xThe identity for tan(x y) has been explained in the following videohttps//youtubYes, sec2 − 1 = tan2x is an identity
Free trigonometric identity calculator verify trigonometric identities stepbystep This website uses cookies to ensure you get the best experience ByVerify the identity 1 cos 2x tan x = sin 2x Use the appropriate doubleangle formulas to rewrite the numerator and dena simplified 1 cos 2x sin 2x 1 Simplify the numerator Enter denominator found in the previ The expression from the previous step then simplifies to tan x using what?Trigonometric Identities and Formulas Below are some of the most important definitions, identities and formulas in trigonometry Trigonometric Functions of Acute Angles sin X = opp / hyp = a / c , csc X = hyp / opp = c / a tan X = opp / adj = a / b , cot X = adj / opp = b / a cos X = adj / hyp = b / c , sec X = hyp / adj = c / b ,
Proving Trigonometric Identities Calculator online with solution and steps Detailed step by step solutions to your Proving Trigonometric Identities problems online with our math solver and calculator Solved exercises of Proving Trigonometric IdentitiesThe double‐angle identity for tangent is obtained by using the sum identity for tangent The half‐angle identity for tangent can be written in three different forms In the first form, the sign is determined by the quadrant in which the angle α/2 is located Example 5 Verify the identityThese identities are sometimes known as powerreducing identities and they may be derived from the doubleangle identity \(\cos(2x)=\cos^2x−\sin^2x\) and the Pythagorean identity \(\cos^2x\sin^2x=1\)



How Do You Verify 4tan 4 Tan 2x 3 Sec 2x 4tan 2x 3 Kinda Hard Please With All The Steps Thanks Socratic


Trig Identities Bingo Card
2 x I started this by making sec 1/cos and using the double angle identity for that and it didn't work at all in any way ever Not sure why I can't do that, but something was wrong Anyways I looked at the solutions manual and they magic out 1 tan x tan 2 x = 1 tanThe more important identities You don't have to know all the identities off the top of your head But these you should Defining relations for tangent, cotangent, secant, and cosecant in terms of sine and cosine The Pythagorean formula for sines and cosines This is probably the most important trig identityCos 2x ≠ 2 cos x;
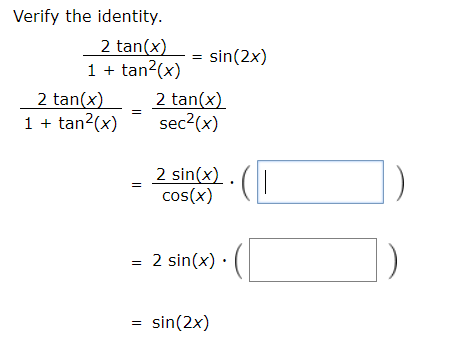


Answered Verify The Identity 2 Tan X 1 Bartleby



How Do You Prove The Identity Tan 2x Secx 1 1 Cosx Cosx Socratic
I need to prove this identity tan^2xsin^2x = tan^2xsin^2x start with left side tan^2xsin^2x =(sin^2x/cos^2x)sin^2xIdentity tan (2x) Multiple Angle Identities Symbolab Identities Pythagorean Angle Sum/Difference Double Angle Multiple Angle Negative Angle Sum to Product Product to SumThese identities can be useful in calculus for converting rational functions in sine and cosine to functions of t in order to find their antiderivatives Technically, the existence of the tangent halfangle formulae stems from the fact that the circle is an algebraic curve of genus 0


Prove Tan 2x Cos 2x Cot 2x Sin 2x 1



Weierstrass Substitution Wikipedia
• Tangent tan 2x = 2 tan x/1 tan2 x = 2 cot x/ cot2 x 1 = 2/cot x – tan x tangent doubleangle identity can be accomplished by applying the same methods, instead use the sum identity for tangent, first • Note sin 2x ≠ 2 sin x;X) 2 1 = 1 cos 2 x There aren't any terms or factors involving in this formula, so let's make up another one An equivalent formula for is math\implies\tan^2x=\dfrac {\sin^2x\sin^2x\cos^2x} {\sin /math Continue Reading2617I'm currently stumped on proving the trig identity below $\tan(2x)\tan (x)=\frac{\tan (x)}{\cos(2x)}$ Or, alternatively written as $\tan(2x)\tan (x)=\tan (x)\sec
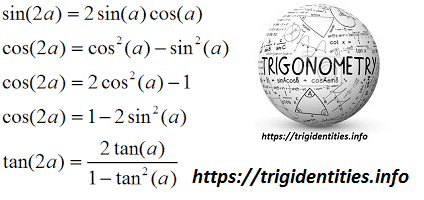


Trig Double Identities Trigonometric Double Angle Functions Trig



How Do You Prove The Identities Cosx Secx Sinx Cscx Sec 2x Tan 2x Socratic
Tan 2x ≠ 2 tan x by Shavana GonzalezIn this video you will learn how to verify trigonometric identitiesverifying trigonometric identitieshow to verify trig identitieshow to verify trigonometricExcellent application of Pythagorean Trig Identities email anilanilkhandelwal@gmailcom



1 Tan 2x 1 Cos 2x Sin 2x 2sin 4x 1 Sin 2x Trigonometric Identities Mcr3u Youtube


Ilectureonline



0 件のコメント:
コメントを投稿